dwarf carnivorous moose per square mile. Approximate the number of dwarf
carnivorous moose in the region 
using the partition given by the lines x = 10 and y = 20, with midpoints
of each rectangle as representatives (xij*, yij*).
1. A field biologist determines that the population density of dwarf
carnivorous moose in a particular region is roughly given by
dwarf carnivorous moose per square mile. Approximate the number of dwarf
carnivorous moose in the region
using the partition given by the lines x = 10 and y = 20, with midpoints
of each rectangle as representatives (xij*, yij*).
The best way to start is with a sketch of the region
and partition lines something like that shown here. The midpoints of each
rectangle (the red dots) have coordinates (5,10), (15,10), (5,30), and
(15,30). Each rectangle is 10 by 20, so has an area of 200 square miles.
Then we put together the sum:
Total = 200M(5,10) + 200M(15,10) + 200M(5,30)
+ 200M(15,30), or 
dwarf carnivorous moose (as some people surmised, some of the moose aren't
full-grown yet).
2. If the Earth is taken to be a sphere 3963 miles in radius and the
atmosphere is 600 miles thick, write a triple integral in spherical coordinates
that would produce the total amount of ozone above the northern hemisphere
given a function 
for the density of ozone per cubic mile.
The region we want lies outside a sphere
of radius 3963, yet within a sphere of radius 3963 + 600 = 4563. To limit
ourselves to the northern hemisphere corresponds to values for phi between
0 and pi/2, and we want all the way around, so theta from 0 to 2pi. The
only other tricky part is to remember to include the appropriate Jacobian,
so the integral should look like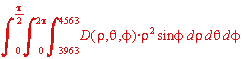 
.
3. Show that the Jacobian for the transformation to spherical coordinates
(
, 
, 
)
is 
.
See example 4 in Stuart section 13.9, p. 886.
Note that little algebra slips were absolutely
not important, and cost a point or two at most -- the outline was what
I was really looking for. The trouble, of course, is that those little
slips could produce dead ends, and there's not much way to help that.
4. Evaluate the integral 
.
This is nearly identical to the second problem
from the quiz. If you try to do the antiderivative as it stands, you get
nowhere. Take that as a clue: Try changing the order of integration and
see if it's better that way.
The
region looks something like what's sketched here, with the curved line
being y = x2. If we reverse the order of integration we'll be
using bottom-to-top slices like the red one instead of left-to-right slices
like the blue one. This means we go from y = 0 on the bottom to y = x2
on the top, and we do this for (red) slices ranging from where x = 0 to
where x = 1.
The new integral is 
.
This is easy to antidifferentiate with respect to y, and when we evaluate
at the limits becomes 
.
This in turn goes well, as using a u = x3 + 1 substitution turns
it into 
.
This antidifferentiates as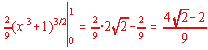 
.
5. Set up limits of integration for 
if the region E is bounded by x = 0, y2 + z2 = 9,
and x = 10 - z. You need not work out the integral.
This is a slight variation on problem 16 from Stewart section 13.7, p. 873.
Imagine
a paper towel tube around the positive x axis (the cylinder y2
+ z2 = 9) cut vertically by the plane x = 0 and diagonally by
the plane x = 10 - z. The easiest way to fill it with strands of spaghetti
is to run them down the length of the tube, from x = 0 to x = 10 - z, the
diagonal plane. Then the limits for x are done, and we just need limits
for y and z that describe the circle in the yz plane. This will produce
an integral something like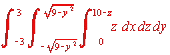 
.
There are also clever ways to do this with cylindrical
coordinates, but they require some real mental flexibility for interchanging
axes or something of that sort -- unless you're sure of what you're doing
it's not likely worth it.
6. Evaluate the integral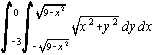 
Clue #1: The antiderivative is awfully hard as it stands.
Clue #2: Lots of things look kind of like circles.
Deduction: Maybe we should switch it to polar coordinates!
The
graph looks something like this, the left half of a circle of radius
3. In polar coordinates, then, the integral becomes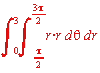 
.
Working this out is pretty routine, giving 9pi.
7. Show that the center of mass of a beautiful purple disk of any radius, centered at the origin and with constant density, is at the origin.
This is essentially problem 39 from the chapter 13 review, except using the whole disk -- and of course in this case the disk is purple.
We need some constant to represent the radius
of the disk -- I'll use R. We also need a letter to represent this constant
density, I'll use D. Then we set up Mx and My, which
is easiest if we use polar coordinates for the round region, so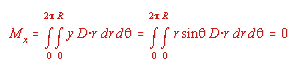 
,
with lots of routine work involved in that last equals sign.
Similarly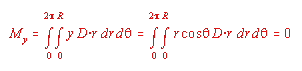 
,
again with lots of work to show that final equals sign holds. Then no matter
what the mass is (so long as it's non-zero) the center of mass is at the
origin.
8.Show that the area of the part of the plane z = ax + by + c that projects
onto a region D in the xy-plane with area A(D) is 
.
This is problem 17 from Stuart section 13.6, p. 865.
The problem is actually easier than it looks at first, but you've got to get started before that becomes apparent, and then take a good look at what you have. For the surface area formula we need our surface's partial derivatives, so we compute zx = a and zy = b. Then we can get as far as setting up the integral (even though we might not yet have any idea how to go from there without any limits of integration),
.Now it's a matter of looking at what we have and
recognizing how close it is to what we want. The radical is exactly part
of what we want to head for, and the only trouble is to find the area of
the region D someplace. Without any concrete description of the region,
all we could do is say it's 
-- but then if we look at what's in front of us, all we need to do is factor
the radical (which is all constant) out of our expression for Surface Area
above, and we have
.So to answer the question about "What good is
it to know that you can do a double integral of 1 to get an area," we can
now answer "Because then you can recognize it as an area when it shows
up in a formula."
9. Buffy is a calculus student at Oklahoma State. She says "Like, our
professor told us that when you use that Fubini thingy, like, all the little
limits switch around, y'know? But, like, y'know, I figured out that it's
the same when you do 
as when you do 
,
so, I mean, I guess it's okay to just switch, like, the dz and the dy,
or whatever, y'know?"
Is Buffy right, or are there corrections or limitations that should be made to her statement?
There were lots of great answers to this, many of them completely different from each other. One good approach was to show Buffy the difference between the two regions her limits described. Another good way to come at it was by talking about why it does work in her example, due to symmetry and the fact that the integrand didn't involve the y or z that she's switching limits on. Other good explanations are possible too.
One way that isn't without drawbacks is just telling her how she should switch the limits. There's nothing wrong with that, but it doesn't really address the question of whether Buffy is right, or why. All that really need to be said on that is that she got lucky in this case, but it won't necessarily work on other problems. It was also nice if an example was provided of very similiar problems where Buffy's move doesn't work, but that's certainly not necessary as long as somehow the point gets across that just because it works once doesn't mean it will always work.
There was a secret bonus point available, of course, for pointing out the other correction that could be made to Buffy's statement -- fixing that split infinitive -- but nobody mentioned it.
By the way, in several years worth of exams with
Buffy problems, nobody has ever hit on Buffy before. The surprises
never end...
10. A truncated paraboloid is formed between the surface z = x2 + y2 and the plane z = a for some positive constant a. To what depth should the paraboloid be filled with water in order that the water have exactly half the volume of the whole solid? (Yes, you need to work out the formula for the volume of the paraboloid even if you remember it from the problem set.)
For a sketch of the derivation of the paraboloid
volume see the Key to Problem Set #2. The key
stuff for us now is that it works out as 
.
So if that's the volume of the whole paraboloid, we want to fill to a depth
d which creates half that volume, so 
.
Solving this for d gives 
.
Extra Credit (5 points possible):
Show why the Jacobian of the transformation x = u(x,y), y = v(x,y) is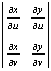 
.
See Stewart, pp. 881-882.