Calculus IV Exam 3 Fall 1998 11/19/98
Each problem is worth 10 points. Show all work for full credit. Please
circle all answers and keep your work as legible as possible. Danger: Not
responsible for losses due to unexpected thawing.
1. Compute 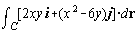 where C is a counterclockwise arc of the ellipse 4x2 + 9y2
= 36 beginning at (3,0) and ending at (0, -2).
where C is a counterclockwise arc of the ellipse 4x2 + 9y2
= 36 beginning at (3,0) and ending at (0, -2).
This was problem #1 from Quiz
4.
Briefly: We can find the potential function f
= x2y - 3y2, and then compute f(0,-2) - f(3,0) =
-12 - 0 = -12.
2. Compute 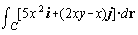 if C is the portion of the parabola y = x2 from (0,0) to (2,4).
if C is the portion of the parabola y = x2 from (0,0) to (2,4).
The mixed partials don't match, so there's no
potential function and we have to do it the hard way.
We parametrize the curve as r(t) = <t,t2>
for 0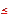 t
t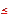 2
(other parametrizations are possible too, of course). Then r'(t)
= <1,2t>. Work out F(r(t)) = <5t2, 2t3-t>,
so our integral dots these and looks like
2
(other parametrizations are possible too, of course). Then r'(t)
= <1,2t>. Work out F(r(t)) = <5t2, 2t3-t>,
so our integral dots these and looks like 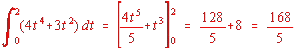 .
.
3. Show that the line integral in problem 2 depends on the path C taken
from (0,0) to (2,4).
There are several good ways to do this. One is
to pick a different path from (0,0) to (2,4), the easiest being a straight
line, and work the line integral along that path to confirm that they're
different (the integral along a straight line should come out to 92/3).
Another way to go, much easier and quicker, is
to rely on some theorems. We know that (as long as the continuity-type
conditions are met, which they are here) a vector field will have a potential
function, and thus be conservative and independent of path, if the mixed
partials are equal. On this field the partial of P with respect to y is
0, whereas the partial of Q with respect to x is 2y - 1, so since they're
different we know the line integrals depend on paths taken.
4. Calculate 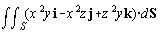 where S is the surface of the rectangular box bounded by the planes
x=0, x=3, y=0, y=2, z=0, and z=1.
where S is the surface of the rectangular box bounded by the planes
x=0, x=3, y=0, y=2, z=0, and z=1.
This was problem #4 in section 14.9 of Stewart.
We wouldn't dream of computing the flux separately
through each of the six sides of this box, there must be a better way.
The Divergence Theorem to the rescue! This will
convert a flux integral through the sides of the box to a triple integral
on the interior of the box. The resulting integral will look like 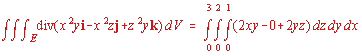 .
The rest is routine and produces a final value, and hence flux through
the box, of 24.
.
The rest is routine and produces a final value, and hence flux through
the box, of 24.
5. When the ice sheet that forms over much of the arctic in winter
breaks up during the spring thaw, occasionally a polar bear ends up floating
off on a small iceberg which might be caught by ocean currents and swept
out away from shore. Suppose this were to happen to a bear named Harvey,
and he floated for weeks until the ice pretty much melted away to a piece
just big enough for him to sit on. Then, unfortunately for Harvey, his
ice raft gets caught in a rare ocean whirlpool effect like the vector field
F = 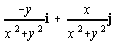 .
Use a line integral to compute the amount of work (i.e.,
.
Use a line integral to compute the amount of work (i.e., 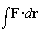 )
for Harvey to paddle his ice raft once around the whirlpool (counterclockwise
of course) along a circle of radius 10 meters.
)
for Harvey to paddle his ice raft once around the whirlpool (counterclockwise
of course) along a circle of radius 10 meters.
It's the usual routine:
I. Parametrize the path. r(t) = <10cos
t, 10sin t>.
II. Work out F(r(t)) = 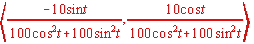 ,
which simplifies nicely to <-sin t / 10, cos t / 10>.
,
which simplifies nicely to <-sin t / 10, cos t / 10>.
III. Work out r'(t) = <-10sin t, 10cos
t>.
IV. Set up the integral -- it's huge at first
but then melts away: 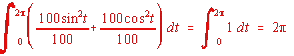 .
.
6. Explain how you can use the fact that curl(grad f) = 0 for
any function f with continuous second partials to conclude that F
= xyi - 2yzj + x3zk does not have a potential
function.
The reasoning goes like this: If F had
a potential function f, then that means grad f = F. Now we know
for any function f (with the usual limitations to reasonably sane functions),
curl(grad f) = 0. But substituting makes this curl(F) = 0.
It's easy to work out the curl of the function given (it's 2yi -3x2zj
- xk), and see that it's not 0. Thus we conclude that F
didn't have a potential function after all.
Summary: If F had a potential function
f, then curl(grad f) = 0, so curl(F) = 0, but it's
not, so it doesn't.
7. Show that if F(x,y,z) is any constant vector field and G(x,y,z)
= xi + yj + zk, then curl(F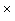 G)
= 2F.
G)
= 2F.
This problem was taken from Ellis & Gulick
2nd, p. 877 #33.
It's mostly just a matter of keeping yourself
oriented through a mean tangle of algebra. We want to show that something's
true of any constant vector field F. Well, any constant vector field
will be of the form ai + bj + ck for some real numbers
a, b, and c, so we take this and cross it with G to get  = (bz-cy)i + (cx-az)j + (ay-bx)k. It's worth noting
at this point that if you arrange that final stuff just right, part of
it spells yak.
= (bz-cy)i + (cx-az)j + (ay-bx)k. It's worth noting
at this point that if you arrange that final stuff just right, part of
it spells yak.
Now we need to work out the curl of this, which
looks like 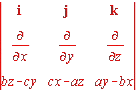 = (a+a)i + (b+b)j + (c+c)k = 2ai + 2bj
+ 2ck = 2(ai + bj + ck) = 2F.
= (a+a)i + (b+b)j + (c+c)k = 2ai + 2bj
+ 2ck = 2(ai + bj + ck) = 2F.
8. Show that the surface area of the helicoid (spiral ramp!) with parametrization
x(u,v) = u cos v, y(u,v) = u sin v, and z = v, within the region S,
is given by  .
.
 We'll
need to use our formula for the surface area of a parametrized surface,
and that requires that we first (duh!) parametrize the surface. It's pretty
much done for us, we just need to write it as r(u,v) = <u cos
v, u sin v, v> so we can work out the partials that the formula calls for,
ru = <cos v, sin v, 0> and rv =
<-u sin v, u cos v, 1>. We need to work out the cross product of these
partials, which is
We'll
need to use our formula for the surface area of a parametrized surface,
and that requires that we first (duh!) parametrize the surface. It's pretty
much done for us, we just need to write it as r(u,v) = <u cos
v, u sin v, v> so we can work out the partials that the formula calls for,
ru = <cos v, sin v, 0> and rv =
<-u sin v, u cos v, 1>. We need to work out the cross product of these
partials, which is  .
This determinant works out to (sin v - 0)i + (0-cos v)j +
(u cos2 v + u sin2 v)k. The coefficient of
k simplifies neatly to u(cos2 v + sin2 v)
= u(1) = u. Now the formula doesn't call for just this product, but rather
for its magnitude, so we work that out:
.
This determinant works out to (sin v - 0)i + (0-cos v)j +
(u cos2 v + u sin2 v)k. The coefficient of
k simplifies neatly to u(cos2 v + sin2 v)
= u(1) = u. Now the formula doesn't call for just this product, but rather
for its magnitude, so we work that out: 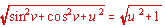 .
Put this inside the formula's integral with the appropriate limits, and
we're done.
.
Put this inside the formula's integral with the appropriate limits, and
we're done.
9. Biff says "So I was doin' this homework for calc, and there's somethin'
I don't get. There's this problem about doin' the line integral of this
function F = 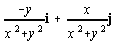 on a circle with radius 2 goin' round the origin. The book says it's 2
on a circle with radius 2 goin' round the origin. The book says it's 2 ,
but I used the Green's thing and got it to be zero. Is the book just wacked
or what?"
,
but I used the Green's thing and got it to be zero. Is the book just wacked
or what?"
As always there are a ton of ways to approach
Biff's problem. Also as always, Biff's done his computations just fine,
and his problem is a little more sophisticated.
One possible response: This is the same function
we integrated in problem #5, and certainly didn't get 0. So we've got two
answers, one from the most basic method and one from the fanciest, and
they don't agree. Which one is more reliable? Well, it's Green's Theorem
that has all the little warning labels about "use only on simple closed
curves" and "if rash persists more than 5 days, see a physician." That's
a good place to be suspicious. And indeed, our function is horribly discontinuous
at the origin, so Green's (which requires not just the function but even
its partials to be continuous) just doesn't apply.
10. Show that the flux of the vector field F(x,y,z) = xi
+ yj + zk outward through a smooth closed surface S
is three times the volume V of the region E enclosed by the
surface.
This problem was taken from Finney/Thomas 1990,
p. 985 #15, although it's really just a very nice generalization of problems
43 and 47 from the chapter 14 review in Stewart..
It's possible to directly compute the flux, but
much easier to use the Divergence Theorem. This turns our problem into 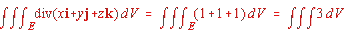 .
If we just realize how much this resembles the formula for the volume of
the region E, we can factor out the 3 and what we have is 3V.
.
If we just realize how much this resembles the formula for the volume of
the region E, we can factor out the 3 and what we have is 3V.
Extra Credit (5 points possible):
The book gave a couple alternate statements of Green's Theorem in the
section on curl and divergence. In one of these the expression 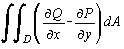 is replaced with
is replaced with 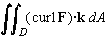 .
Show that these are equivalent (Remember F = Pi + Qj
+ 0k).
.
Show that these are equivalent (Remember F = Pi + Qj
+ 0k).
It's in the book, Stewart p. 928.
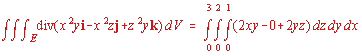 .
The rest is routine and produces a final value, and hence flux through
the box, of 24.
.
The rest is routine and produces a final value, and hence flux through
the box, of 24.
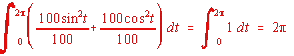 .
.
 = (bz-cy)i + (cx-az)j + (ay-bx)k. It's worth noting
at this point that if you arrange that final stuff just right, part of
it spells yak.
= (bz-cy)i + (cx-az)j + (ay-bx)k. It's worth noting
at this point that if you arrange that final stuff just right, part of
it spells yak.
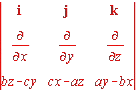 = (a+a)i + (b+b)j + (c+c)k = 2ai + 2bj
+ 2ck = 2(ai + bj + ck) = 2F.
= (a+a)i + (b+b)j + (c+c)k = 2ai + 2bj
+ 2ck = 2(ai + bj + ck) = 2F.
 We'll
need to use our formula for the surface area of a parametrized surface,
and that requires that we first (duh!) parametrize the surface. It's pretty
much done for us, we just need to write it as r(u,v) = <u cos
v, u sin v, v> so we can work out the partials that the formula calls for,
ru = <cos v, sin v, 0> and rv =
<-u sin v, u cos v, 1>. We need to work out the cross product of these
partials, which is
We'll
need to use our formula for the surface area of a parametrized surface,
and that requires that we first (duh!) parametrize the surface. It's pretty
much done for us, we just need to write it as r(u,v) = <u cos
v, u sin v, v> so we can work out the partials that the formula calls for,
ru = <cos v, sin v, 0> and rv =
<-u sin v, u cos v, 1>. We need to work out the cross product of these
partials, which is  .
This determinant works out to (sin v - 0)i + (0-cos v)j +
(u cos2 v + u sin2 v)k. The coefficient of
k simplifies neatly to u(cos2 v + sin2 v)
= u(1) = u. Now the formula doesn't call for just this product, but rather
for its magnitude, so we work that out:
.
This determinant works out to (sin v - 0)i + (0-cos v)j +
(u cos2 v + u sin2 v)k. The coefficient of
k simplifies neatly to u(cos2 v + sin2 v)
= u(1) = u. Now the formula doesn't call for just this product, but rather
for its magnitude, so we work that out: